One of the most non-intuitive consequences of Einstein’s theory of relativity is that there is no absolute space or absolute time. If you ask where and when you are, you will answer. But if you and I disperse a long distance, and I ask you where and when, as you think I am, then our answers with you will not necessarily match. It turns out that in the general theory of relativity there is no universal method for determining space and time (and distances) except where you are. As a result, we have many ways to identify such things, and the reader’s question is related to this:
I would like to see your explanation of conformal time and accompanying distance - what it is, when and how they are used compared to the usual time and distance.
Using concepts such as “time” and “distance” in ordinary speech, we make many assumptions that we rarely think about.

If you think that you can tell me that where I am, the time of day is 10:05 am, and I am 700 meters away from you, you may not understand on what basis you are confident in your assessments. You assume that our clocks go with the same speed, that they come from the same place where we agreed on the value of time, and that when we put these clocks together again, they will also be coordinated with each other. It's simple, isn't it?
But this is possible only if two important conditions are met:
1. Nothing moves in relation to everything else. If two objects acquire speed relative to each other, they experience the passage of time (and the sense of distance) in different ways. Unstable particles moving at speeds close to the speed of light seem to us to live longer due to time stretching, and astronauts onboard the ISS, moving rapidly around the Earth, age a little differently than people who are stationary on Earth.
2. The space is absolutely flat, which never happens. In the Universe, GR works, and according to it, the existence of matter and energy means that space is curved, and that clocks go at different speeds depending on the depth of immersion in the gravitational field. The clock at the top of the Empire State Building each year is a few microseconds behind the clock at its foot.
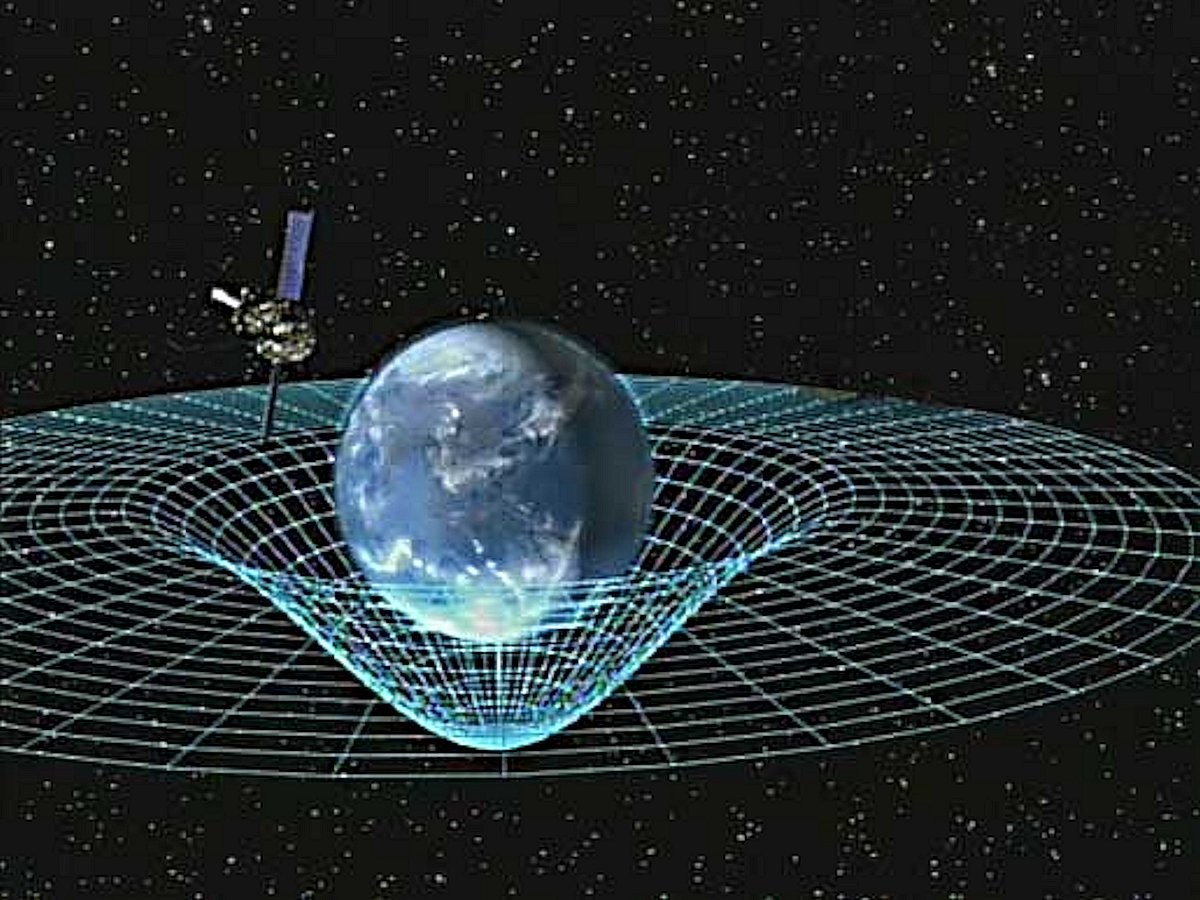
The same restrictions also apply to distances: the movement and curvature of space make it impossible for observers in different places to accept the universal standard of distances. But for really large distances, something else begins to play a role: the fact of the expansion of the very fabric of the space of the Universe on cosmic scales. We can no longer speak of the distances between the galaxies as that which can be measured with a certain consistent ruler, since the space between the galaxies expands with time. And this leads to problems when we start talking, for example, about the most distant galaxies in the Universe.

The current space record holder for distances is located at a red shift of 11.1, which means that during the existence of the Universe, 13.8 billion years from the Big Bang, its light reached us, having been on the way 13.4 billion years. But how far away is this galaxy from us? You could, on the basis of the time spent on the journey, decide that it is 13.4 billion light-years away — but that is hardly the case. When the light that reached us was emitted by this galaxy, it was no more than two billion light years from us. Thanks to the expansion of the universe, using the generally accepted standard of measurement, we can say that it is now 32 billion light years away. The universal standard of distance is hard to define in an expanding universe, in which distances change over time.

So one of the concepts we introduced when answering a reader's question is the concept of different types of distances. He asks us about one of them - about the
accompanying distance . This is one of my favorite concepts: it implies that distances in the Universe change due to the Hubble expansion, so it excludes expansion from calculations. This is very convenient for simulating the formation of such structures of the Universe as stars, galaxies, clusters and filaments. Gravity, of course, does its bit, but the Universe has been expanding all this time. Knowing how to adjust the distances for expansion, we can see how large-scale structures of the Universe evolve. Visually, it is much more interesting to observe this than to follow the expansion of the Universe and try to discern the structure formation process in all of this.
Since space and time are inextricably linked to the concept of space-time that unites them, we need a new concept of time, corresponding to each of the new concepts of distance, which we will invent. The temporal partner of the concomitant distance is conformal time. If we could magically instantly freeze the entire expansion of the Universe in all places, then the conformal time corresponds to how much the light beam will take time to go from a certain place to you.
For the most distant galaxy in the Universe, the conformal time will be 32 billion years. For perceived distance from the Big Bang, it will be 46 billion years. And this is despite the fact that 400 million years passed from the Big Bang to the emission of light by the first galaxy. In the early stages, the expansion of the Universe was so fast — and the result is still felt today — that the difference of 14 billion years of conformal time corresponds to the difference of 400 million years of “correct” time (the one we call simply “time”).
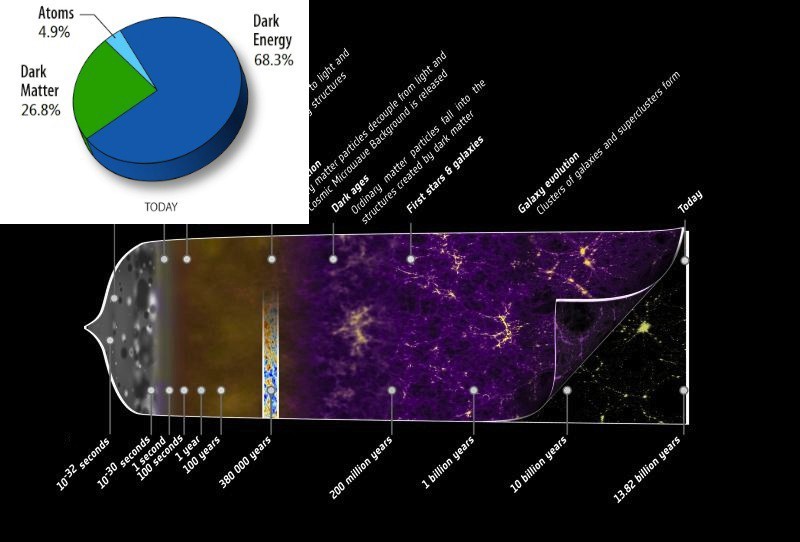
If we talk about events that take place on Earth, where nothing moves with speeds close to light and does not change too much in the gravitational field, then the different types of “distances” and “times” will coincide. But if we talk about an expanding Universe on a cosmic scale, then the correct distance and the correct time may not be as useful and interesting as the accompanying distance and conformal time. And the next time you see a simulation of the Universe and see that the Universe does not look expanding, keep in mind that the simulation takes place using the accompanying distances, although it can use the correct time.
And when you hear something about a very distant object that is less than 14 billion light-years from us, keep in mind that most likely it is about the accompanying distance. According to our usual, correct lines, this distance is likely to be much greater!