An eminent mathematician revealed the details of how his success in studying thousands of years of mathematical questions related to concepts taken from physics
 Minion Kim
Minion KimMathematics is full of weird number systems that most people have never heard of. Some of them will even be difficult to imagine. But
rational numbers are familiar to all. These are numbers for counting objects and fractions - all numbers known to us from elementary school. But in math it is sometimes harder to understand the simplest things. They are simple, like a smooth wall, without cracks and protrusions, or other obvious properties that could be grasped.
Minyon Kim , [
Minhyong Kim ] a mathematician from the University of Oxford, is particularly interested in the question of which rational numbers are suitable for solving equations of a certain kind. This problem has stimulated specialists in number theory for millennia. And they barely advanced on the way to its solution. When a question has been studied for so long and without an answer, it can be concluded that the only way to advance in it will be to push a radically new idea. That's exactly what Kim did.
“The technician is not so much, although we have been working on it for the last 3000 years. So when someone presents a truly new way to do this, it is of great interest - and Mignon did just that, ”says Jordan Ellenberg, a mathematician at the University of Wisconsin-Madison.
Over the past decade, Kim has described a completely new way of finding patterns in the seemingly disorderly world of rational numbers. He described this method in articles and at conferences, and handed it over to students, who now continue this work independently. But he always saved something. His vision, which gave life to his ideas, was not based on a pure world of numbers, but on concepts borrowed from physics. For Kim, rational decisions are a bit like the trajectory of light.
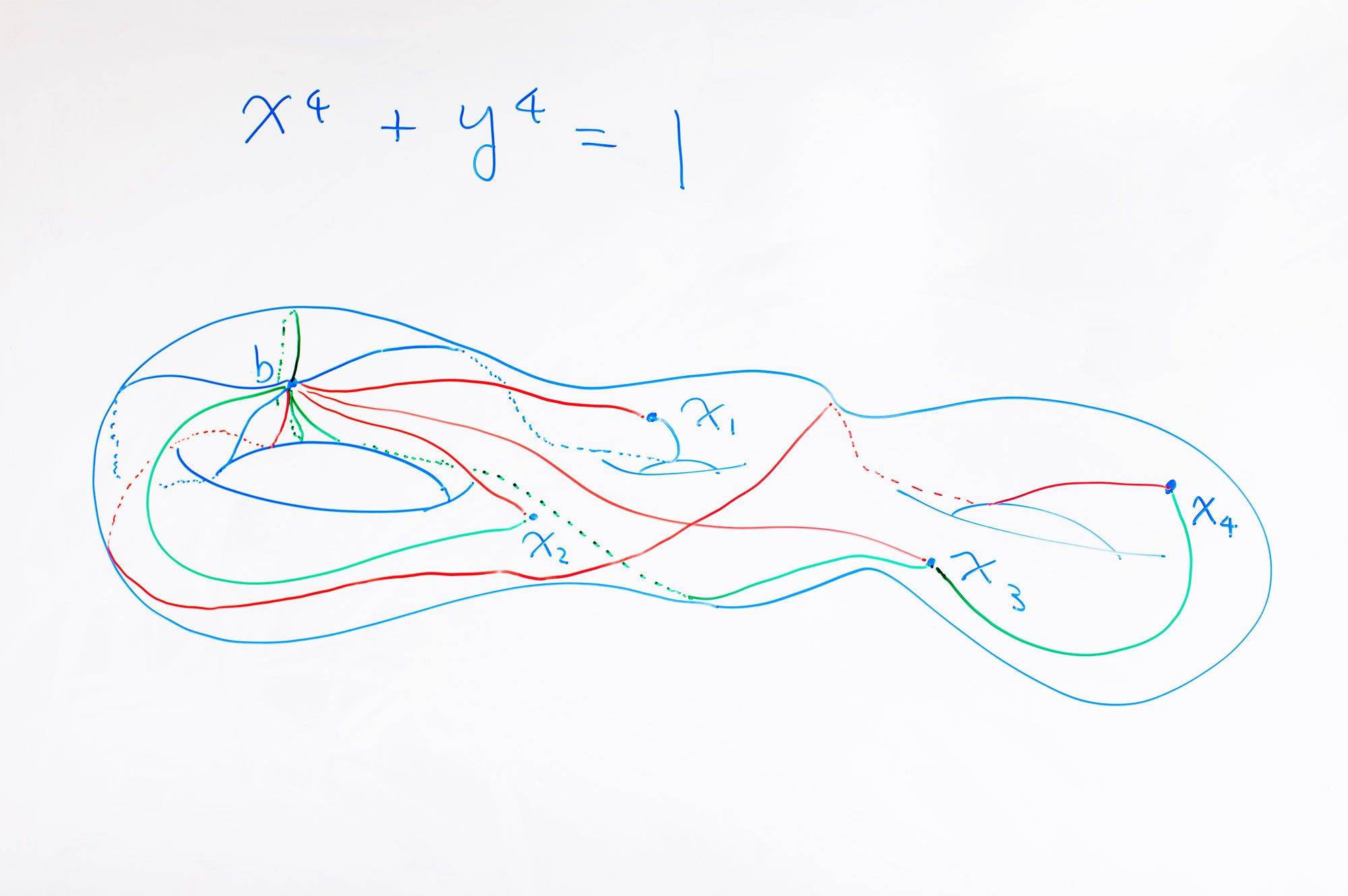 A mathematical object, a three-hole torus, adorns Kim's board at Oxford University.
A mathematical object, a three-hole torus, adorns Kim's board at Oxford University.If this relationship seems fantastic, then it is, even for mathematicians. Therefore, Kim did not disclose her details for a long time. “I hid it, because for many years I was confused by the connection with physics,” he says. “Number theory specialists are very practical people, and the influence of physics sometimes makes them skeptical of mathematics.”
But now Kim says he is ready to share his vision. “I think that change is just a symptom of aging!” Kim, who turned 53, wrote in one of the first letters we exchanged to write this story.
He recently held a conference that brought together number theory and string theory experts. He also sketched out drafts of articles that begin to describe his inspiration for the mathematical community, who are not used to thinking about numbers using such direct analogies with the physical world.
There remains only one obstacle - the last part of the analogy of physics in mathematics, which Kim has yet to work through. He hopes that by inviting others to share his vision, especially physicists, he will receive the help needed to complete the work.
Ancient mystery
Rational solutions of equations actively attract the human mind. They bring satisfaction, similar to what you get from the pieces of the puzzle that have come into place. Therefore, they are the heroes of the most famous mathematical hypotheses.
Rational numbers include integers and any number that can be expressed as a ratio of two integers, for example, 1, -4, or 99/100. Mathematicians are especially interested in such rational numbers that solve Diophantine equations - polynomial equations with integer coefficients, for example, x
2 + y
2 = 1. They are named after the mathematician
Diophantus , who studied them in Alexandria in the 3rd century AD
Rational solutions are difficult to find in the general case, since they do not obey any geometric regularity. Take the equation x
2 + y
2 = 1. Its solutions in
real numbers form a circle. Remove all points on the circle that cannot be expressed as a fraction, and only rational decisions will remain that do not form such an accurate object. Rational solutions look randomly scattered around the circle.
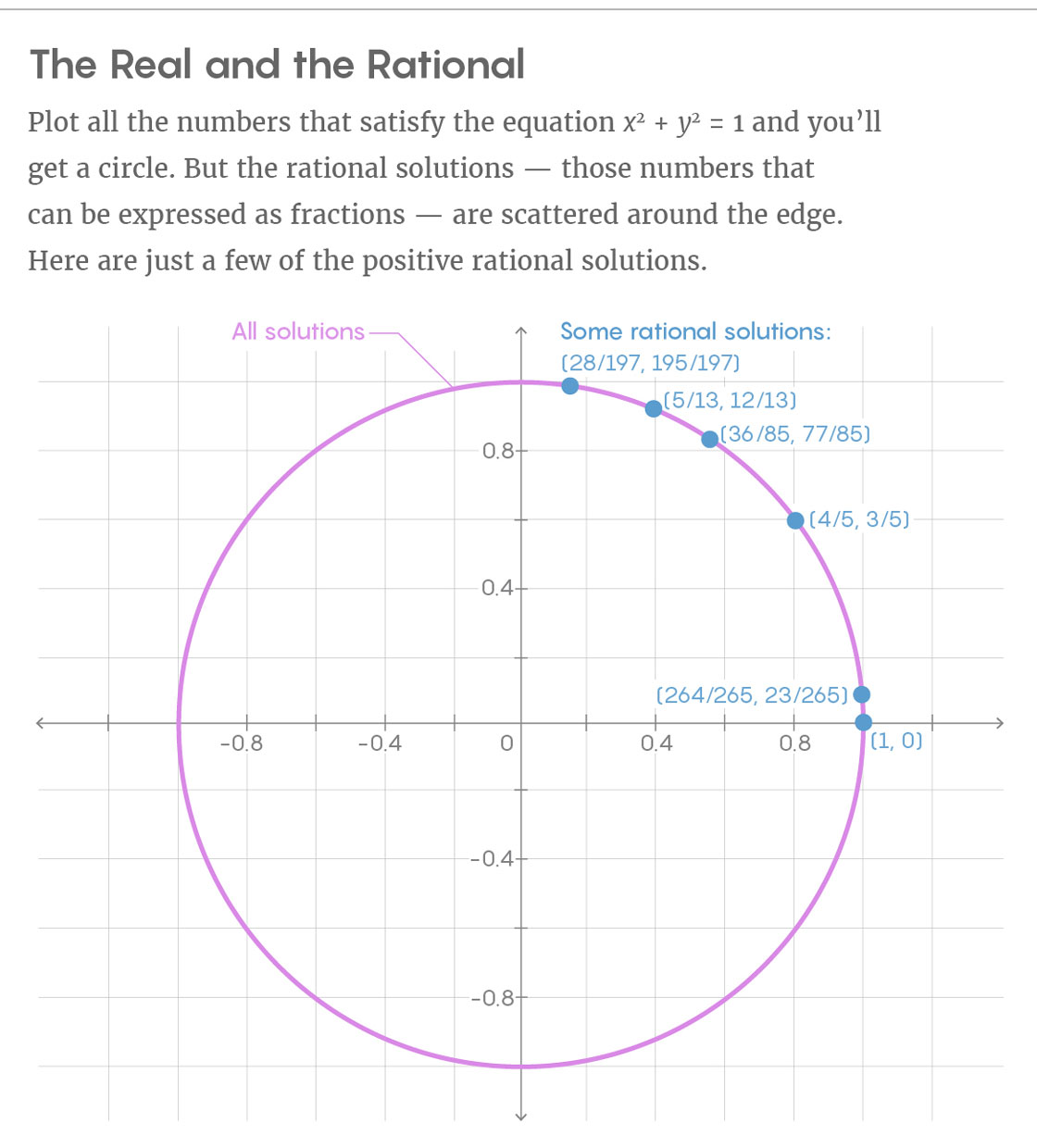
“The condition that a point must obey in order to have rational coordinates is not at all geometric. It’s impossible to write an equation that rational points must satisfy, ”says Kim.
It is often quite simple to find one rational solution, or even several. But it is more interesting for mathematicians who do not like incompleteness to find all rational solutions. And it is much more difficult. It is so difficult that the proof of even a small statement about the number of rational solutions is enough to be considered a mathematical star. In 1986,
Gerd Faltings won the
Fields Premium , the highest math award, mainly for proving
the Mordell hypothesis , which says that certain classes of Diophantine equations have only a finite number of rational solutions.
The Faltings proof was the turning point of number theory. And also by the fact that mathematicians call “ineffective proof”, because it did not give the exact number of rational solutions, and did not find them. Since then, mathematicians have been looking for ways to take these next steps. Rational points appear random on a regular equation graph. Mathematicians hope that by changing the context in which they reflect on the problem, you can make these points look like a meaningful combination that can be described in some precise way. The problem is that well-known areas of mathematics do not provide such a context.
 Kim in his office in Oxford
Kim in his office in Oxford“To obtain effective results from rational points, there is clearly a need for a new idea,” said Ellenberg.
Now there are two main assumptions about the nature of such an idea. One comes from the Japanese mathematician
Shin-ichi Mochizuki , who in 2012 published on the page of his faculty at Kyoto University a
complex and innovative mathematical work of several hundred pages. After five years, this work remains largely incomprehensible. Another new idea came from Kim, who tried to imagine rational numbers in an expanded numeric context, in which their hidden patterns begin to appear.
Symmetrical solution
Mathematicians often say that the more symmetrical an object is, the easier it is to study it. Therefore, they would like to place the study of Diophantine equations under conditions containing more symmetry than those where this problem arises naturally. If this works out, they can use the newly acquired symmetries to track the rational points they need.
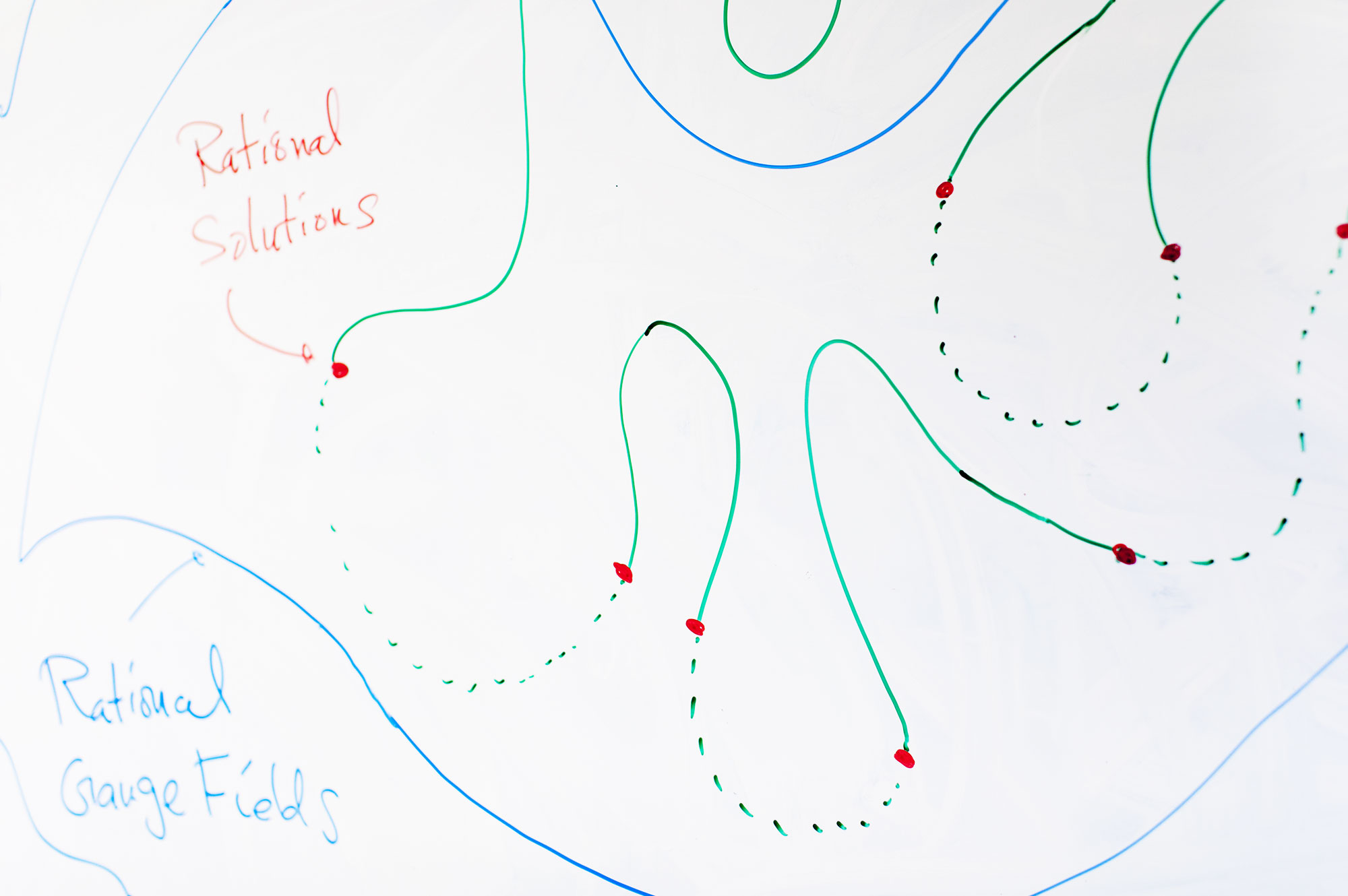
To understand how symmetry helps math to orient in a problem, imagine a circle. Perhaps your goal is to identify all the points on the circle. Symmetry helps a lot because it creates a map that allows you to move from points you know to those that are still to be discovered.
Imagine finding all the rational points at the bottom of a circle. Since the circle has a mirror symmetry, the points can be reflected up (changing the signs of all the coordinates of y) and suddenly all points from the upper part can be obtained. The circle is generally so rich in symmetry that to find all points on it you need to find out the position of only one point and then combine it with knowledge of circle symmetry: you just need to apply the infinite rotary symmetry of the circle to the starting point.
But if the geometric object with which you work is much less correct, such as, for example, a randomly wagging path, you will have to work very hard to define each point separately - you will not have symmetrical relations that allow you to mark unknown points with help known.
Numeric sets can also have symmetries, and the more symmetries in the set, the easier it is to understand - you can apply symmetric relationships to detect unknown values. Numbers with certain kinds of symmetry form a "group", and mathematicians use the properties of a group to understand the numbers it contains.
Many rational solutions of an equation need not have symmetry and do not form a group, which is why mathematicians face an unreal task in trying to find solutions one by one.
From the 1940s, mathematicians began to study methods for arranging Diophantine equations in more symmetrical conditions. Mathematician Claude Chaboti [Claude Chabauty] discovered that inside a larger geometric space created by him (using the expanded universe of numbers called
p-adic numbers ), rational numbers form their own symmetric subspace. He took this subspace and
combined it with the graph of the Diophantine equation. The points of their intersection turned out to be rational solutions of the equation.
In the 1980s, mathematician
Robert Coleman clarified the work of Chaboti. Over the next couple of decades, the Coleman-Chaboti approach was the best mathematical tool that mathematicians had for finding rational solutions to Diophantine equations. But it works only in the case when the graph of the equation corresponds with the size of a larger space in a certain proportion. When it is violated, it becomes difficult to accurately find the position of the intersection points of the equation curve and rational numbers.
“If your curve is in the surrounding space where there are too many rational points, they start to accumulate and it’s hard to distinguish which of them are on the curve,” says Kiran Kedlaya, a mathematician at the University of California at San Diego.
And here comes Kim. In order to expand the work of Chaboti, he wanted to find an even larger space in which one can investigate Diophantine equations - a space where rational numbers are more fragmented, which allows studying intersection points with a large number of varieties of Diophantine equations.


Space spaces
If you need a larger space and some hints about how you can use symmetry to navigate in it, physics will do for you.
In the general case, space in the mathematical sense is any set of points with a geometric or topological structure. Thousands of points scattered willy-nilly, do not form space - they are not connected together by structure. But the sphere, which is a connected organization of points, is already a space. Like the torus, or two-dimensional plane, or four-dimensional space-time, where we live.
In addition to these, there are even more exotic spaces that can be imagined as “spaces of spaces”. The simplest example: let's say you have a triangle - and this space. Now imagine the space of all possible triangles. Each point in it represents a certain triangle, and the coordinates of this point are given by the angles of the triangle represented by it.
Such an idea is often useful in physics. In the framework of the general theory of relativity, space and time are constantly evolving, and physicists consider each configuration of space and time as a point in the space of all configurations of space-time. Space spaces also appear in the field, which physicists call gauge invariance, working with fields superimposed on physical space. These fields describe how forces such as electromagnetism and gravity change when moving in space. One can imagine that at each point in space the configuration of these fields is slightly different - and that all these different configurations form points in the “space of all fields” of a higher dimension.
This field space from physics is an analogy close to Kim's sentence for number theory. To understand this, imagine a ray of light. Physicists represent light moving through the space of fields of higher dimension. In this space, the light will follow a path that adheres to the principle of least resistance and minimizes the amount of time it takes to get from point A to point B. This principle explains why light bends when moving from one material to another - a curved path minimizes temporary expenses.
Larger spaces of spaces, appearing in physics, possess additional symmetries, which are absent in any of the spaces represented by them. These symmetries attract attention to certain points, for example, focusing on the way that minimizes time. The same symmetries, constructed in a different way and in a different context, can be focused on other points — for example, points corresponding to rational solutions of equations.
Connecting symmetry with physics
In number theory, there are no particles that can be tracked, but there is something like spacetime in it, and it offers a way to draw paths and create space of all possible paths. From this basic correspondence, Kim develops schemes in which the “task of finding the trajectory of light and finding rational solutions to Diophantine equations are two facets of one problem,” he explained at a conference on mathematical physics last week in Heidelberg.
Solutions of Diophantine equations form spaces - these are curves defined by equations. These curves can be one-dimensional, like a circle, or multi-dimensional. For example, if you build a complex solution of the Diophantine equation x
4 + y
4 = 1, you get a torus with three holes. Rational points on such a torus do not have a geometric structure — that is why they are hard to find — but they can be compared with points in a multidimensional space of spaces that have such a structure.
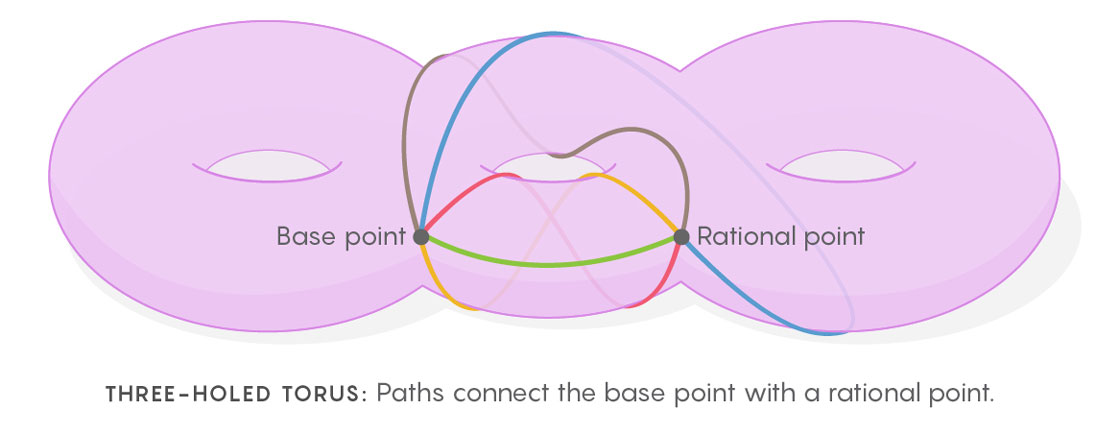
Kim creates this multidimensional space of spaces, presenting the ways in which you can draw closed curves on the torus (or in the space that defines the equation). The procedure for drawing curves looks like this. First you need to select the starting point, then draw a loop from this point to any other, and return to the first. Now repeat this process, drawing the paths connecting the base point with all the other torus points. You will get a thicket of all possible loops starting and ending at the base point. This set of loops is a centrally important object of mathematics, called the
fundamental group of space.
Any point on the torus can be used as a starting point. Each point will have a unique thicket of paths outgoing from it. Each of these collections of paths can be represented as a point in the multidimensional "space of all sets of paths" (as the space of all possible triangles). This space of spaces is geometrically very similar to what physicists build in gauge invariance theory: how sets of paths change when you go from one point on a torus to another strongly resembles how fields change when you go from one point to another in real space. This space of spaces has additional symmetries that are not on the torus itself. And although rational points on the torus have no symmetry, if we go into the space of all sets of paths, we can find symmetries between the points associated with rational numbers. You acquire symmetries that were not visible before.
“I sometimes say that the hidden arithmetic symmetry is coded in these paths, which is very similar to the internal symmetries of the gauge invariance theory,” said Kim.
Like Chaboti, Kim finds rational solutions by examining intersection points in the larger space they create. It uses the symmetry of this space to come to the points of intersection. He hopes to develop an equation that precisely defines these points.
In a physical context, one can imagine all the possible ways in which a ray of light can go. This is your “space of all paths”. Physicists are interested in points of this space that correspond to time-minimizing paths. Kim believes that the points corresponding to the thickets of the paths coming from rational points have something like the same property - that is, these points minimize some property that arises when you consider the geometric forms of Diophantine equations. He just has not figured out yet what this property might be.
“What I began to try to find,” is the principle of least resistance in a mathematical context, he wrote to me in a letter. “I haven’t found it yet, but I’m sure it exists.”
Uncertain Future
Over the past few months, I have described Kim's vision, inspired by physics, several mathematicians, fans of his contributions to number theory. But having learned about such details of his work, they were lost.
“As a theorist of representative values, if I were shown all those amazing achievements made by Mignon and asked if they were inspired by physics, they would say: What the hell are you?” Said Ellenberg.
So far, Kim did not mention physics in any of his works. Instead, he writes about objects called "Selmer variations", and considers the relationship between Selmer variations in the space of all Selmer variations. Such things are familiar to number theory specialists. But for Kim, they were always just another designation of certain physical objects.
“There must be a way to use physical ideas to solve problems in number theory, but we haven’t thought out enough yet how to create such a platform,” said Kim. “We are in such a state when our understanding of physics is quite well developed, and quite a lot of number theory specialists are interested in it to take the next step.”
The main obstacle for Kim's method is to look for some action to minimize the space of all sets of loops. In the physical world, this approach looks natural, but in arithmetic there is no obvious meaning. Even the mathematicians who closely follow Kim's work are not sure if he can find it.
“I think Kim’s program will do a lot of great things for us. I don’t think that we will have as clear as Mignon wants, an understanding of whether rational points are classical solutions to arithmetic gauge theory, ”says Arnav Tripathy, a professor of mathematical physics at Harvard University.
Today the language of physics practically does not overlap with the practice of the theory of numbers. Kim believes that this will almost certainly change. Forty years ago, physics, geometry, and topologies had little in common. Then, in the 1980s, several mathematicians and physicists, now representing significant figures, found precise ways to use physics to study the properties of forms. After this development, this area has changed and no longer returned to the old methods.
“Nowadays it is almost impossible to be interested in geometry and topology, without knowing anything about physics. I’m pretty sure that this will happen with number theory in the next 15 years, Kim said. "All connections are extremely natural."